The diagram was constructed with straightedge and compass tools, a topic that has fascinated mathematicians, architects, and artists for centuries. These simple yet powerful tools have enabled the creation of intricate geometric designs, precise technical drawings, and even the exploration of fundamental mathematical concepts.
In this comprehensive guide, we will delve into the historical significance of straightedge and compass tools, explore the geometric principles that underpin their use, and provide step-by-step instructions for constructing various geometric shapes. We will also discuss the practical applications of these tools in design and architecture, and highlight their educational value in promoting spatial reasoning and problem-solving skills.
Historical Context
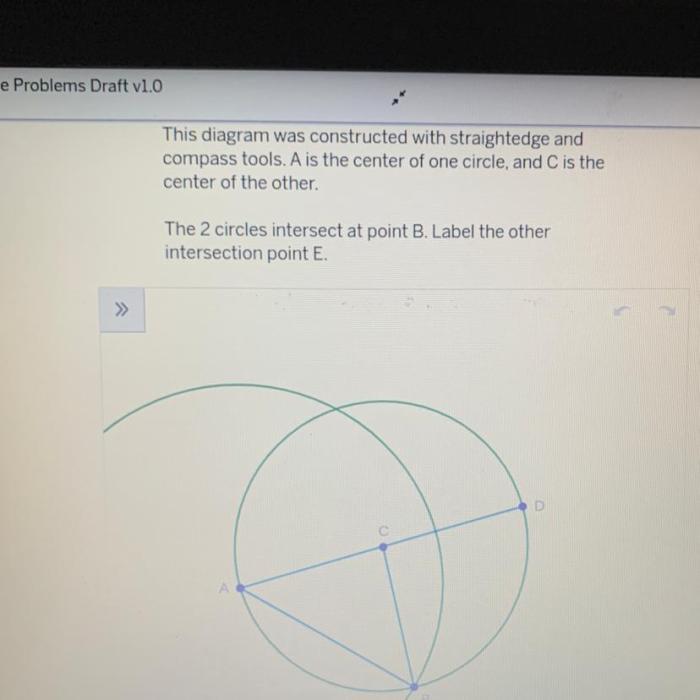
Straightedge and compass tools have played a significant role in geometry since ancient times. These tools allowed early mathematicians to construct precise diagrams and figures, enabling the development of geometric principles and theorems. The use of straightedge and compass tools in ancient geometry laid the foundation for advancements in architecture, engineering, and other fields.
Some famous diagrams constructed using these tools include the Pythagorean theorem, which demonstrates the relationship between the sides of a right triangle, and the Golden Ratio, a geometric proportion found in nature and art.
Geometric Principles
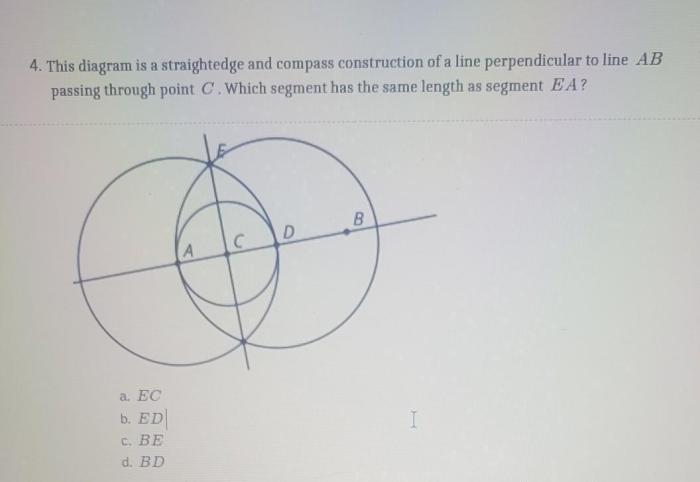
The construction of diagrams with straightedge and compass tools relies on fundamental geometric principles. These tools allow for the precise creation of lines, circles, angles, and other geometric elements. The straightedge is used to draw straight lines, while the compass is used to draw circles and arcs.
By combining these basic elements, it is possible to construct more complex geometric shapes, such as triangles, squares, and polygons. The relationships between these shapes and their angles, sides, and areas are governed by geometric principles, which can be demonstrated and explored using straightedge and compass tools.
Methods and Techniques
Creating Lines
To draw a line using a straightedge, simply place the straightedge on the desired location and draw along its edge.
Creating Circles, The diagram was constructed with straightedge and compass tools
To draw a circle using a compass, set the compass to the desired radius and place the point on the center of the circle. Then, rotate the compass around the center to draw the circle.
Creating Angles
To create an angle using a straightedge and compass, first draw two lines that intersect at the desired vertex. Then, place the compass on the vertex and set it to the desired angle measure. Rotate the compass to draw the angle.
Applications in Design and Architecture: The Diagram Was Constructed With Straightedge And Compass Tools
Straightedge and compass tools are essential for creating precise plans, blueprints, and other technical drawings in design and architecture. These tools allow architects and engineers to accurately lay out the dimensions, angles, and shapes of buildings, structures, and other objects.
In addition, straightedge and compass tools are used in landscape architecture to design gardens, parks, and other outdoor spaces. The ability to create precise geometric shapes and patterns is crucial for creating aesthetically pleasing and functional outdoor environments.
Educational Value
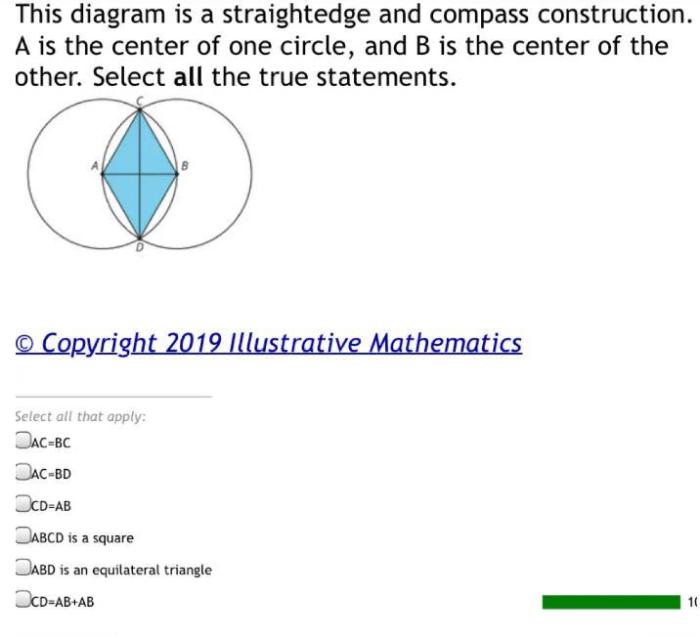
Using straightedge and compass tools in geometry education promotes spatial reasoning, problem-solving skills, and an understanding of geometric concepts. By constructing diagrams and figures, students can visualize and explore geometric relationships in a hands-on way.
Straightedge and compass tools also encourage students to think critically and creatively. They must apply geometric principles and techniques to solve problems and create accurate diagrams. This process helps students develop their logical thinking and problem-solving abilities.
General Inquiries
What are the fundamental principles of geometry that underlie the construction of diagrams with straightedge and compass tools?
The fundamental principles of geometry that underlie the construction of diagrams with straightedge and compass tools include the axioms of Euclidean geometry, such as the postulates of congruence and similarity. These principles allow for the precise creation of geometric shapes and figures, based on the properties of points, lines, angles, and circles.
What are some practical applications of straightedge and compass tools in design and architecture?
Straightedge and compass tools are used in design and architecture to create precise plans, blueprints, and other technical drawings. They are essential for ensuring the accuracy and consistency of geometric constructions, which is crucial for the structural integrity and aesthetic appeal of buildings and other structures.
What is the educational value of using straightedge and compass tools in geometry education?
Using straightedge and compass tools in geometry education promotes spatial reasoning, problem-solving skills, and an understanding of geometric concepts. By constructing geometric shapes and figures, students develop a deeper understanding of their properties and relationships, and learn to apply geometric principles to real-world problems.